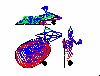Finite-element modelling of the cat middle ear
with elastically suspended malleus and incus
W.R.J. Funnell
Poster presentation at 19th Midwinter Res. Mtg.,
Assoc. Res. Otolaryngol., St. Petersburg Beach (1996)
Abstract
Previous models of the cat middle ear have assumed the existence of a fixed axis of
rotation, corresponding to the classical notion of an axis through the anterior process
of the malleus and the posterior process of the incus. Recent experimental
measurements of manubrial motion have made it clear that the axis of rotation is not
fixed, especially at high frequencies but to a considerable extent even at lower
frequencies. Precise three-dimensional measurements of the complex frequency-dependent motion of the cat manubrium have recently become available (Decraemer
et al., 1994; Decraemer & Khanna, 1996).
This paper extends our finite-element model of the cat middle ear to include elastic
suspensions of the malleus and incus, in place of the previously fixed axis of rotation.
Highly simplified representations are used for the attachment of the anterior mallear
process to the temporal bone and for the ligament which attaches the posterior incudal
process to the temporal bone. The geometry is based on a three-dimensional
reconstruction from serial histological sections.
A range of plausible values is used for the stiffnesses of the suspensions of the
malleus and incus. The resulting three-dimensional motion of the manubrium is
presented for comparison with experimental measurements.
Supported by MRC Canada.
1. Introduction
Our previous models of the cat middle ear have assumed the
existence of a fixed axis of rotation, corresponding to the
classical notion of an axis through the anterior process of the
malleus and the posterior process of the incus. Recent
experimental measurements of manubrial motion have made it
clear that the axis of rotation is not fixed, especially at high
frequencies but to a considerable extent even at lower
frequencies. Precise three-dimensional measurements of the
complex frequency-dependent motion of the cat manubrium
have recently become available (Decraemer, Khanna & Funnell,
1994; Decraemer & Khanna, 1996).
This poster presents a preliminary extension of our finite-element model of the cat middle ear to include elastic
suspensions of the malleus and incus, in place of the previous
fixed axis of rotation. Highly simplified representations are used
for the attachment of the anterior mallear process to the
temporal bone and for the ligament which attaches the posterior
incudal process to the temporal bone. The geometry is based on
a three-dimensional reconstruction from serial histological
sections.
2. 3-D reconstruction from serial sections
 We have created a 3-D reconstruction of a cat middle ear,
based on a set of histological sections obtained from S.M.
Khanna (Columbia University). Outlines of selected structures
were manually digitized and aligned. Fig. 1 shows the eardrum
and ossicles, colour coded as follows:
We have created a 3-D reconstruction of a cat middle ear,
based on a set of histological sections obtained from S.M.
Khanna (Columbia University). Outlines of selected structures
were manually digitized and aligned. Fig. 1 shows the eardrum
and ossicles, colour coded as follows:
gray tympanic membrane
red malleus
green incus
blue stapes
yellow soft tissues
 Fig. 2 shows a part of
one of the histological
sections. The structures
outlined are the wall of the
middle-ear cavity; the body
of the tensor tympani
(upper left); the head and
anterior process of the
malleus, joined by a thin web; the posterior process of the incus;
and the two bundles of the posterior incudal ligament (lower
right).
Fig. 2 shows a part of
one of the histological
sections. The structures
outlined are the wall of the
middle-ear cavity; the body
of the tensor tympani
(upper left); the head and
anterior process of the
malleus, joined by a thin web; the posterior process of the incus;
and the two bundles of the posterior incudal ligament (lower
right).
3. Finite-element model
The finite-element model presented here includes shell
representations of the eardrum, malleus, incus and stapes, plus
springs representing the annular ligament, cochlear load, and
suspensions of the malleus and incus. The eardrum part of the
model is essentially the same as we have used previously
(Funnell et al., 1987, 1992). The models of the stapes and
cochlear load have been described previously by Ladak &
Funnell (1993, 1994). The incudostapedial joint is represented
simply by 8 triangular shell elements forming a block (Ghosh &
Funnell, 1995).
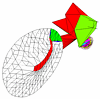
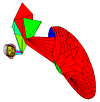 The hollow shell models used here for the malleus and incus
are new. Two views of the model are shown in Figs. 3 and 4
.
The Figures are colour coded as for Fig. 2. The yellow lines
represent spring elements corresponding to the soft tissues and
the cochlear load.
The hollow shell models used here for the malleus and incus
are new. Two views of the model are shown in Figs. 3 and 4
.
The Figures are colour coded as for Fig. 2. The yellow lines
represent spring elements corresponding to the soft tissues and
the cochlear load.
If the lateral bundle of the posterior incudal ligament is
approximated by a cube with edges of 0.5 mm, and its Young's
modulus E is taken to be 200 Mdyn cm-2, then the use of the
formula AE/l gives an axial stiffness of 10 Mdyn cm-1. The
medial bundle is about the same width but only about a third as
thick, so its stiffness has been estimated to be 30 Mdyn cm-1.
For each bundle, the stiffness has been divided between two
spring elements attached to the end of
the posterior incudal process, as shown in Fig. 3. This provides
both translational and rotational stiffness.
In our histological material the anterior process of the
malleus appears to be attached to the wall of the middle-ear
cavity by a large but thin layer of amorphous material. This has
been crudely represented by using a spring at each corner of a
triangular element representing the thin bony web between the
neck and anterior process of the malleus, as shown in Fig. 4. For
a surface area of 2 mm2, a thickness of 0.1 mm, and a small
Young's modulus of 2 Mdyn cm-2, the total stiffness is
4 Mdyn cm-1, to be divided among the three springs.
4. Results
Fig. 5 displays contour lines representing the low-frequency
displacement amplitudes computed for the model for a uniform
sound pressure of 100 dB SPL. Fig. 6 shows the displacements
as vectors. The maximal displacement of the eardrum (729 nm),
the relative displacement of the manubrium, and the position
and orientation of the apparent axis of rotation, are all fairly
similar to results obtained with our earlier fixed-axis model and
to experimental results. The axis of rotation runs roughly
parallel to the expected line from the anterior mallear tip to the
posterior incudal tip.
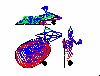 Fig. 5. Displacement-amplitude contours. (a,b,c) z, x, and y
components of displacement, viewed along the z, x, and y axes,
respectively. Green and red contours represent displacements
out of and into the page, respectively. Black contours
correspond to zero displacement. The contour spacings are
normalized to the maximum value for each component: (a) 729
nm; (b) 103 nm; (c) 175 nm.
Fig. 5. Displacement-amplitude contours. (a,b,c) z, x, and y
components of displacement, viewed along the z, x, and y axes,
respectively. Green and red contours represent displacements
out of and into the page, respectively. Black contours
correspond to zero displacement. The contour spacings are
normalized to the maximum value for each component: (a) 729
nm; (b) 103 nm; (c) 175 nm.
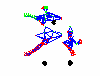 Fig. 6. Displacement vectors. (a,b,c) Views along the z, x and y
axes, respectively. Green arrows point out of the page, red
arrows point into the page. The arrow lengths are normalized
to the overall maximum component value of 296 nm.
Fig. 6. Displacement vectors. (a,b,c) Views along the z, x and y
axes, respectively. Green arrows point out of the page, red
arrows point into the page. The arrow lengths are normalized
to the overall maximum component value of 296 nm.
Figs. 7 and 8 show the model's displacements when the
stiffnesses are all either decreased or increased by a factor of 2,
and then by a factor of 100. When the stiffnesses are halved and
doubled (Fig. 7), the maximal drum displacement changes by
+9% and -12%, respectively; the orientation and position of the
axis of rotation change somewhat but the axis still runs
anteroposteriorly within the bodies of the malleus and incus.
When the stiffnesses are decreased by a factor of 100 (Fig. 8a)
the drum displacement increases by only 23% but the axis of
rotation shifts superiorly almost to the top of the head of the
malleus. When the stiffnesses are increased by a factor of 100
(Fig. 8b) the drum displacements decrease by 58% and there is
practically no rotation of the ossicles.
 Fig. 7. Displacement-amplitude contours.
(a) Model stiffnesses halved. (b) Model stiffnesses doubled.
Fig. 7. Displacement-amplitude contours.
(a) Model stiffnesses halved. (b) Model stiffnesses doubled.
 Fig. 8. Displacement-amplitude contours.
(a) Model stiffnesses divided by 100.
(b) Model stiffnesses multiplied by 100.
Fig. 8. Displacement-amplitude contours.
(a) Model stiffnesses divided by 100.
(b) Model stiffnesses multiplied by 100.
5. Conclusions
The model presented here includes a rough approximation for
the geometry of the cat malleus and incus, and of the soft tissue
suspending them from the wall of the middle-ear cavity, and
plausible estimates for the material properties. The model
produces a low-frequency displacement pattern and an axis of
rotation which are similar to experimental observations.
Variation of the stiffness parameters by a factor of 100 results
in unrealistic displacements. Variation by a factor of 2 results in
changes that are large enough to be significant for quantitative
comparisons with experiments on individual cats.
6. References
1. Decraemer, W.F., Khanna, S.M., & Funnell, W.R.J. (1994): A method for
determining three-dimensional vibration in the ear. Hearing Res. 77: 19-37
2. Decraemer, W.F., & Khanna, S.M. (1996): Three dimensional vibrations of the
malleus in the cat middle ear. Assoc. Res. Otolaryngol. Midwinter Mtg., paper
#780
3. Funnell, W.R.J., Decraemer, W.F., & Khanna, S.M. (1987): On the damped
frequency response of a finite-element model of the cat eardrum. J. Acoust.
Soc. Am. 81(6): 1851-1859
4. Funnell, W.R.J., Khanna, S.M. & Decraemer, W.F. (1992): On the degree of
rigidity of the manubrium in a finite-element model of the cat eardrum. J.
Acoust. Soc. Am. 91(4): 2082-2090
5. Ghosh, S.S., & Funnell, W.R.J. (1995): On the effects of incudostapedial joint
flexibility in a finite-element model of the cat middle ear. Proc. IEEE EMBS
17th Annual Conference & 21st Can. Med. & Biol. Eng. Conf., Montréal:
1437-1438
6. Ladak, H.M., & Funnell, W.R.J. (1993): Finite-element modelling of the
reconstructed cat middle ear. Proc. 19th Can. Med. & Biol. Eng. Conf.: 370-371
7. Ladak, H.M., & Funnell, W.R.J. (1994): Finite-element modelling of malleus-stapes and malleus-footplate prostheses in cat. 17th Midwinter Res. Mtg.,
Assoc. Res. Otolaryngol., St. Petersburg Beach
Converted from WordPerfect 5.1 to HTML by
R. Funnell
Last modified: Thu, 2001 Jul 26 15:53:53
 We have created a 3-D reconstruction of a cat middle ear,
based on a set of histological sections obtained from S.M.
Khanna (Columbia University). Outlines of selected structures
were manually digitized and aligned. Fig. 1 shows the eardrum
and ossicles, colour coded as follows:
We have created a 3-D reconstruction of a cat middle ear,
based on a set of histological sections obtained from S.M.
Khanna (Columbia University). Outlines of selected structures
were manually digitized and aligned. Fig. 1 shows the eardrum
and ossicles, colour coded as follows: